Student App
Smart & Fun
Matific is serious about making math fun. Students progress on their personalized learning path, complete assigned work and learn through multiplayer activities.
Math Activities for K-6
The World’s Best
Thousands of math activities covering the K-6 curriculum, designed by education experts. From conceptual understanding to fluency, Matific has you covered.
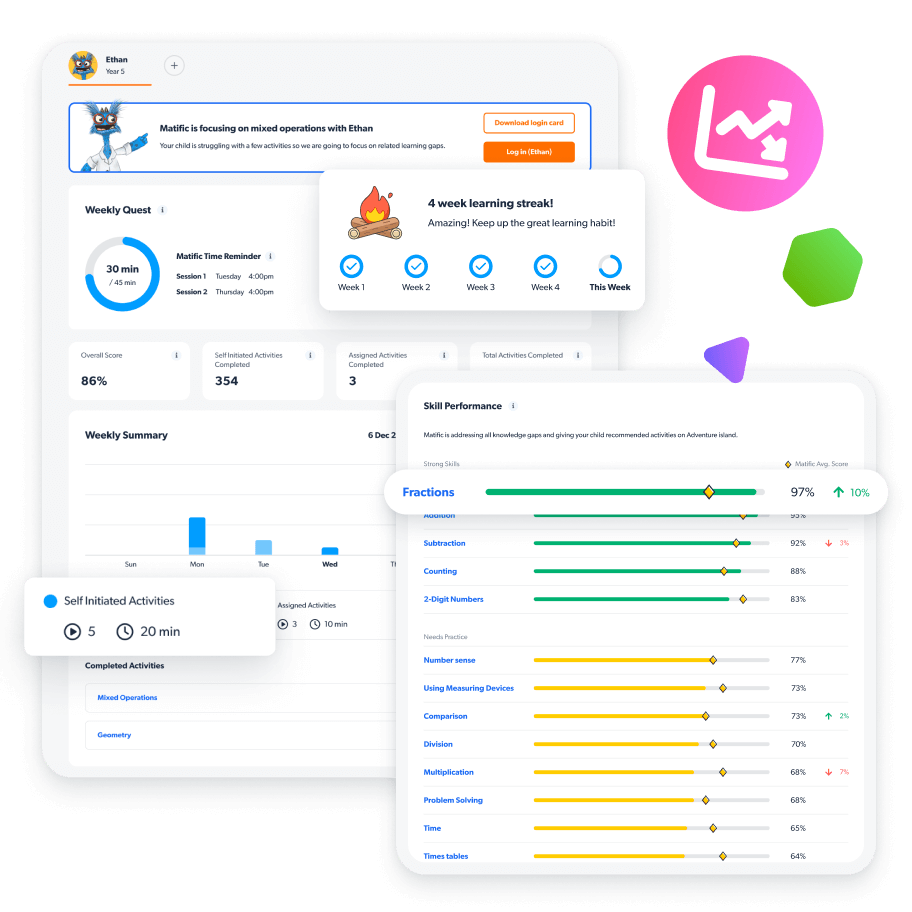
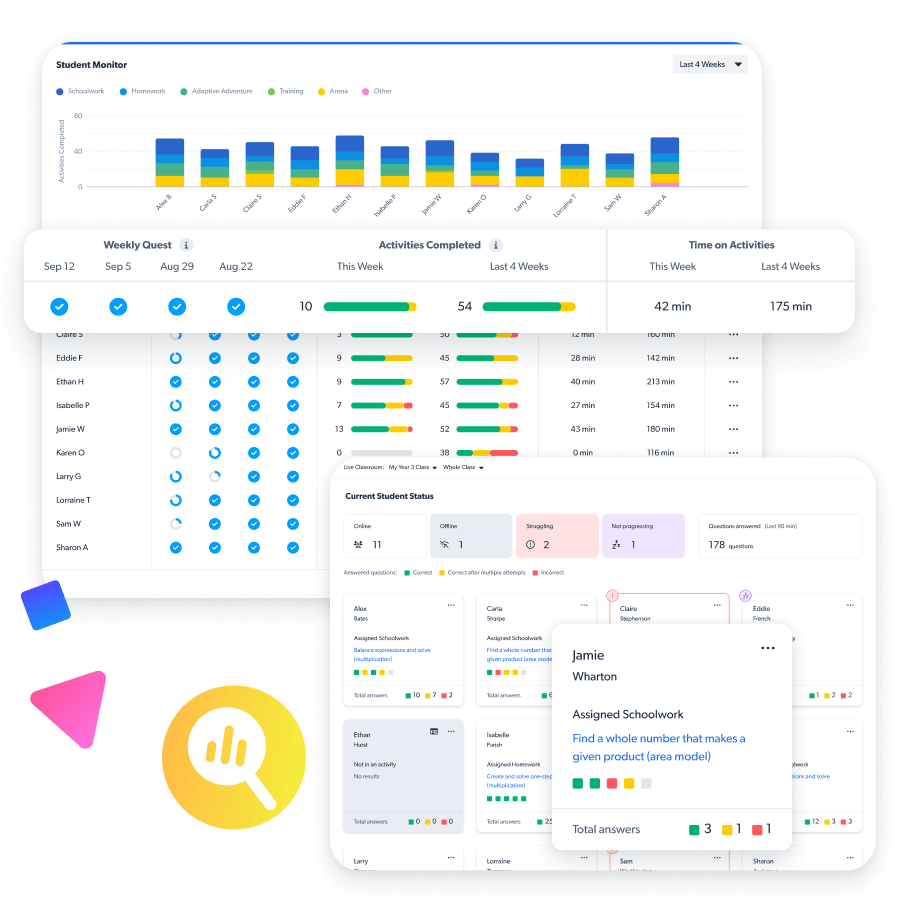
Educator & Parent Tools
Simple yet Powerful
Educators and parents have access to powerful tools that provide control, insights and allow for intervention.
A personalized & adaptive learning path
Mathematics activities for year K-6 with a rigorous pedagogy designed by education experts.
Matific Works

Matific's pedagogical principles
Matific's core strength is our 5-point pedagogical principles developed by experts from Stanford, Harvard, Berkley and the Einstein Institute.
Fully integrated into your Education EcoSystem
Trusted by millions of users worldwide
“Matific is a fantastic tool for teaching mathematics intuitively and introducing children to scientific thinking.”

“Far more engaging for our students than other online platforms and we like how the questions were adaptive to the students' abilities.”

“Matific is very easy to use, and our students enjoyed using it. Parents were also very impressed by what their children were learning and using.”

Matific is your learning companion - anytime, anywhere
-
Available on
-
App Store
-
Google Play
-
Web Browser